Fade Duration

The mobile Rayleigh or Rician radio channel is characterized by rapidly changing channel characteristics. As the amplitude of a signal received over such a channel also fluctuates, the receiver will experience periods during which the signal can not be recovered reliably. If a certain minimum (threshold) signal level is needed for acceptable communication performance, the received signal will experience periods of

sufficient signal strength or "non-fade intervals", during which the receiver can work reliably and at low bit error rate
insufficient signal strength or "fades", during which the bir error rate inevitably is close to one half (randomly guessing ones and zeros) and the receiver may even fall out of lock.

It is of critical importance to the performance of digital mobile networks that the block length or packet duration is chosen taking into account the expected duration of fades and non-fade intervals. One of two approaches can taken:

make the block length at least an order of magnitude longer than the average fade / non-fade period, and rely on error correction to cope with burst errors. This approach can be used for mobile reception of digital broadcast signals (e.g. DAB), particularly if the effect of fading is mitigated through using a wide transmission bandwidth and appropriate signal processing. This approach would be impractical in indoor office communication (wireless LANs) with high bit rates and extremely small Doppler spreads, i.e., with very long fade / non-fade periods.
make the block length shorter than the average fade / non-fade period and retransmit lost data. This approach works best in full duplex mobile data systems and random access data systems.

If the data block length is larger than the average non-fade period, almost all blocks will experience a signal fade and a corresponding burst of bit errors. This may result in an excessive packet dropping rate, unless powerful error correction codes are used. If the system supports a feedback signal with acknowledgments of received blocks, it is mostly advantageous to use only limited error correction coding, but to rely on retransmission of lost blocks. To minimize the number of retransmissions, one should choose the block length shorter than the average fade and non-fade period.

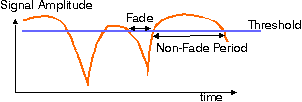
Figure: fade and non-fade duration for a sample of a fading signal.

Average Fade Duration

We use:

Outage Probability = Average number of fades per second * Average fade duration

where the average number of fades per second is called the threshold crossing rate.

Expressions for Average (Non-) Fade Duration

In a Rayleigh fading channel with fade margin M, the average nonfade duration (ANFD) is

              SQRT(M)
ANFD  =   --------------
          SQRT(2 pi) f_D

where f_D is the Doppler spread. M is the ratio of the local-mean signal power and the minimum (threshold) power needed for reliable communication.

Average non-fade duration in Rayleigh-fading channel versus fade margin for n = 1, 2, 3, 4, 5 and 6 Rayleigh-fading interfering signals. Normalized by dividing by the Doppler Spread.

The curve for n = 6 closely resembles the curve the ANFD in an interference-free but noise-limited channel.

Thus

The ANFD is proportional to the speed of the mobile user. Channel fading occurs mainly because the user moves. If the user is stationary almost no time variations of the channel occur (except if reflecting elements in the environment move)
The ANFD increases proportional with the square root of the fade margin.
The non-fade duration is not so sensitive to whether the signal experiences fades below a constant noise-floor or a fading interfering signal.

Calculation of the distribution of non-fade periods is tedious, but has been elaborated by Rice. Because of the shape of the Doppler spectrum, fade durations that coincide with a motion of about half a wavelength are relatively frequent.

The average fade duration (AFD) is

              SQRT(M)
AFD   =   -------------- [ exp{- M} -1]
          SQRT(2 pi) f_D

Thus

The AFD is proportional to the speed of the mobile user.
The fade durations rapidly reduce with increasing fade margin, but the time between fades increases much slower.

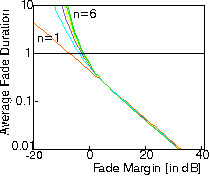
Average fade duration in Rayleigh-fading channel versus fade margin for n = 1, 2, 3, 4, 5 and 6 Rayleigh-fading interfering signals. Normalized by dividing by the Doppler Spread.