www.WirelessCommunication.NL

Chapter: Wireless Channels
Section: Path Loss

Propagation over a Plane Earth

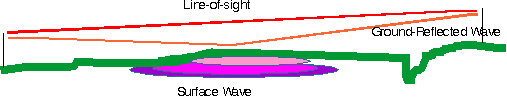
If we consider the effect of the earth surface, the expressions for the received signal become more complicated than in case of free space propagation. The main effect is that signals reflected off the earth surface may (partially) cancel the line of sight wave.

Model

For (theoretical) isotropic antennas above a plane earth, the received electric field strength is

with R_c the reflection coefficient and E_0 the field strength for propagation in free space. This expression can be interpreted as the complex sum of a direct line-of-sight wave, a ground-reflected wave and a surface wave. The phasor sum of the first and second term is known as the `space wave'.

For a horizontally-polarized wave incident on the surface of a perfectly smooth earth,


where epsilon_r is the relative dielectric constant of the earth, Psi is the angle of incidence (between the radio ray and the earth surface) and x = sigma/(2 pi f_c epsilon_0) with sigma the conductivity of the ground and epsilon_0 the dielectric constant of vacuum.

For vertical polarization



Exercise

Show that the reflection coefficient tends to -1 for angles close to 0. Verify that for vertical polarization, abs(R_c) > 0.9 for Psi < 10 degrees. For horizontal polarization, abs( R_c) > 0.5 for Psi < 5 degrees and abs( R_c) > 0.9 for Psi < 1 degree.

UHF Mobile Communication

The relative amplitude F(.) of the surface wave is very small for most cases of mobile UHF communication (F(.) << 1). Its contribution is relevant only a few wavelengths above the ground. The phase difference between the direct and the ground-reflected wave can be found from the two-ray approximation considering only a Line-of-Sight and a Ground Reflection. Denoting the transmit and receive antenna heights as h_T and h_R, respectively, the phase difference can be expressed as


For large d, one finds, using

the expression


,

For large d, (d >> 5h_T h_R ), the reflection coefficient tends to -1, so the received signal power becomes

For propagation distances substantially beyond the turnover point

,

this tends to the fourth power distance law:


Exercise

Discuss the effect of path loss on the performance of a cellular radio network. Is it good to have signals attenuate rapidly with increasing distance?
Answer.

Egli's Model (1957)

 Experiments confirm that in macro-cellular links over smooth, plane terrain, the received signal power (expressed in dB) decreases with "40 log d". Also a "6 dB/octave" height gain is experienced: doubling the height increases the received power by a factor 4.

In contrast to the theoretical plane earth loss, Egli measured a significant increase of the path loss with the carrier frequency f_c. He proposed the semi-empirical model

i.e., he introduced a frequency dependent empirical correction for ranges 1< d < 50 km, and carrier frequencies 30 MHz < f_c < 1 GHz.

For communication at short range, this formula looses its accuracy because the reflection coefficient is not necessarily close to -1. For d << h_T h_R / 4 lambda, free space propagation is more appropriate, but a number of significant reflections must be taken into account. In streets with high buildings, guided propagation may occur.

.

www.WirelessCommunication.NL © Jean-Paul M.G. Linnartz, 1993, 1995.