| Wireless CommunicationChapter: Wireless Propagation Channels |
Contributed by Peter E. Leuthold and
Pascal Truffer
A channel sounder (such as ECHO 24) delivers a
series of complex CIR 
or 
,
respectively, evoked by the wave component impinging from different
directions 
. The extraction of the data which is
necessary to calculate the parameters of the target functions used in
the SRCM represents a fastidious task. Numerous algorithms are
available which can be used to determine either single wave component
parameters or at once a collection of them with satisfying precision.
The important wave component parameters are as follows:
complex envelope (amplitude 
and phase 
)
delay incidence direction 
Doppler shift
A global channel parameter is the number M of active paths which should also be known in order to assure the efficiency of the algorithms.
A crucial task is the determination of the incidence directions of the wave components. Beamforming and Fourier algorithms [10] are widely applied techniques to estimate the angle of arrival. In the past decade, high resolution algorithms have been developed to extract the azimuth and even the delay of the impinging waves. They are based on spectral or non-parametric estimation principles like ESPRIT (Estimation of Signal Processing via Rotational Invariance Techniques) [11] and MUSIC (Multiple Signal Classification) [12]. The latter algorithm can be extended to resolve not only the delay and the incidence direction but also the Doppler shift.
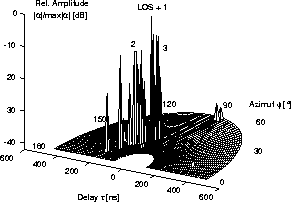
Figure: Estimated PDDP by means of the SAGE algorithm in a
real propagation environment at 2 GHz
Recently, the SAGE (Space-Alternating Generalized
Expectation-Maximization) algorithm has been successfully applied for
the combined estimation of , , , and
[13]. SAGE is an iterative
procedure based on maximum likelihood (ML) estimation and allows the
reduction of the multidimensional optimization problem to a sequence
of problems with lower dimensionality. Figure 9
shows the result obtained by means of the SAGE algorithm at 2 GHz in
the real propagation environment mapped in Figure 10.
The reflections can be clearly identified: LOS and some minor echoes
from the roof in area 1, reflections from the wall of a large concrete
building 2 and echoes from the roof of a staircase 3. The receiver Rx
had been equipped with a linear antenna array consisting of 19
monopole elements in equal intervals of 
.
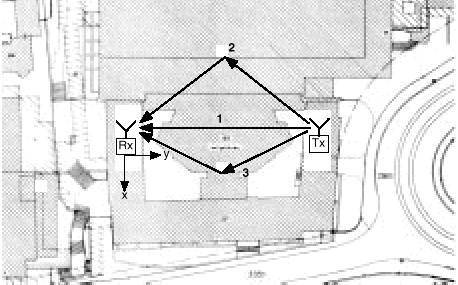
Figure: Map of the environment with the receiver (Rx) and
transmitter (Tx) location
To determine the number M of active paths is a rather difficult problem, too. Considering Figure 2 a first approach seems to be the introduction of a certain power level and then the enumeration of the exceeding maxima. A more detailed investigation of the DScF properties shows that this simple principle would often fail. Thus, algorithms have been developed making use of special weighting procedures [14] which yield satisfying results.
